
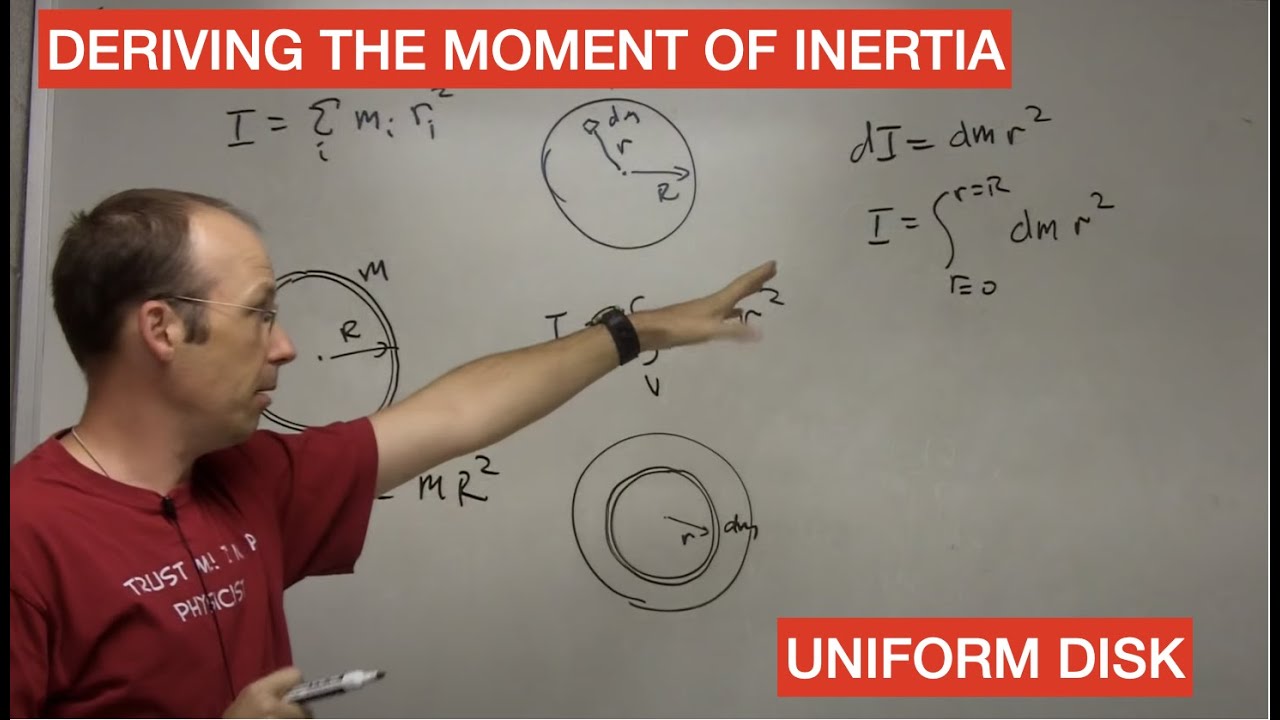
In this case the expression above evaluates to I = 0.4 MR 2, where M is the mass of the sphere and R is its radius.Įven homogeneous materials have some measureable compression as internal pressure increases with depth. Which is independent of the choice of axis as long as it goes through the center of mass.Ī convenient reference moment of inertia is that for a planet having constant density with depth. Expressed as either a discrete sum or integral over a continuous distribution of mass, moment of inertia is given by The moment of inertia is always defined with respect to a specific axis of rotation. Expressed similar to Newton's 2nd law of linear motion, where the time derivative of momentum is equal to the sum of forces acting on a mass, the time derivative of angular momentum is equal to the sum of torques acting on the rotating body. The angular momentum of a body rotating at constant angular velocity is the product Iω, where I is the moment of inertia about an axis of rotation about its center of mass and ω is the angular velocity of rotation. The linear momentum of a mass m moving with constant velocity v is the product mv. The moment of inertia for a body rotating about any axis through its center of mass is the essentially the analog of mass for a body in linear motion. The effects of moment of inertia on the torques needed to change the rotation of a body are similar to the effects of mass on the force needed to change linear motion.


 0 kommentar(er)
0 kommentar(er)
